Using Star Counts to Study the Galaxy

Question: what is the spatial distribution of stars around us?

Measuring distance to many stars is difficult and time consuming.  Let's instead start by relying on the easily observable quantity: the brightness of stars. If we choose stars which are all the same absolute magnitude, we can use their apparent magnitude as a substitute for distance. So let's look at star counts as a function of apparent magnitude.
 
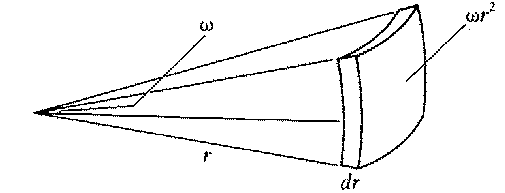
Imagine looking at stars in a patch of sky of solid angle  and at a distance r.

The volume of space in the thin patch between r and r+dr is


 
 
If the galaxy has a uniform density of stars (given by n), and we integrate over radius, we get the total number of stars between us and r:


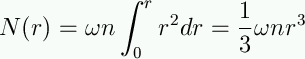Now remember the relationship between absolute and apparent magnitude...


...which we can turn around to solve for r...


...and plug into N(r) to get N(m), the number of stars brighter than some apparent magnitude m:


or:

So for every magnitude fainter we go, we ought to see 100.6 = 4 times as many stars. We don't.

But it gets worse. Let's look at how much light we'd be seeing from these stars.
 
 
Let's say the apparent brightness of an m=0 star is l0. Then, using the definition of magnitudes, the light coming from a star of apparent magnitude m is: 


so the total amount of light coming from stars of magnitude m is:

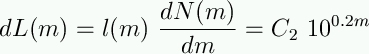So the total amount of light coming from all stars brighter than apparent magnitude m is:

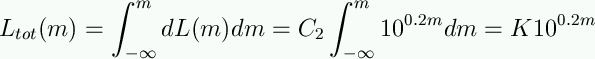
This diverges as m gets bigger: infinite brightness!

This problem is known as Olber's paradox. If the galaxy were infinite and homogeneous, the sky should be blazingly bright.

So what's the point of this failed exercise? It's not a failure! Turn the question around: fit star counts to different models of stellar distributions to derive the structure of the galaxy.