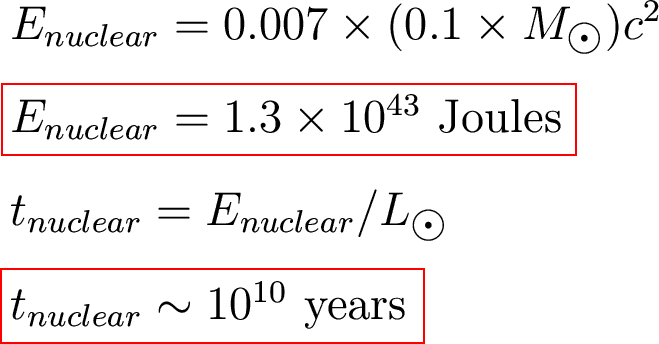Nuclear Reactions
So if gravity can't power the sun, how about
processes inside an atom? We have two choices here:
1. Chemical reactions
(ie reactions dealing with atomic bonds between atoms and
electrons)
- Typical energy per atom of around 10
electron volts
(the amount of energy stored in the atomic levels of hydrogen
and helium).
- Not enough energy to power the Sun for very
long (less
than gravity!)
2. Nuclear reactions (reactions between atomic nuclei)
- Typical energies are
millions
of times larger than for chemical reactions.
- Lots of energy
available!
So time for a little NUCLEAR PHYSICS (woo-hoo!)
Inside the Atom
Let's look at Hydrogen and Helium (98% of the Sun)
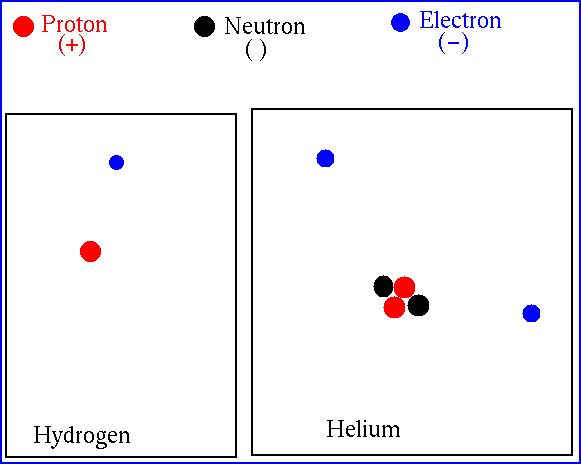 At the center of the Sun, the temperature is high
enough that Hydrogen and Helium (and just about everything else)
is ionized -- the electrons
are no longer bound
(attached) to the atoms. We just deal with bare nuclei.
At the center of the Sun, the temperature is high
enough that Hydrogen and Helium (and just about everything else)
is ionized -- the electrons
are no longer bound
(attached) to the atoms. We just deal with bare nuclei.
Let's do a few definitions to make life easier.
First define the atomic
mass unit
as being 1/12 of the mass of the carbon-12 atom:
1 AMU = 1.660540x10-27
kg
Next, bring  Einstein into the picture. Einstein realized that E=mc2. Mass
and energy are equivalent, related by the speed of light, c.
Einstein into the picture. Einstein realized that E=mc2. Mass
and energy are equivalent, related by the speed of light, c.
So we can also talk about mass in terms of
energy:
1 AMU =~ 931.5
million electron
Volts (MeV)
(where 1 eV = 1.6x10-19 Joules)
Okay, now how does ionization work? If we add
energy to
the atom, we break apart the electron from the proton. Let's
look at
this from a mass perspective.
Since a hydrogen atom is simply a proton plus and
electron, the mass of the hydrogen atom should simply be equal
to the mass if the proton
plus the mass of the electron, right?
Wrong!
M(hydrogen) - M(proton) - M(electron) = -13.6 eV
What? What is this energy difference? It is the binding energy of the hydrogen
atom.
In other words the correct (schematic) equation
is not
hydrogen
= proton + electron
But rather
hydrogen
+ energy = proton + electron
What about nuclear
processes?
Instead of ionizing atoms, let's look at fusing
atoms together.
A hydrogen nucleus is simply a proton.
A helium nucleus is two protons and two neutrons.
We can make helium by fusing together 4 hydrogen
atoms. But look:
4 x M(hydrogen) - M(helium) = 26.71 MeV.
Compare this to what happened when we ionized
hydrogen:
- The energy difference is MeVs, not eVs.
Much bigger.
- The energy left over is positive: energy is produced.
So this time our schematic
equation is
hydrogen + hydrogen
+ hydrogen + hydrogen = helium +
energy
So every time you fuse 4
hydrogen
atoms together to make helium, 26.7 MeV is released. This is
equivalent to
about 0.7% of the mass of the 4 original hydrogen atoms.
But is it enough to
power the
Sun?
Let's estimate how long the
Sun could
shine by fusing hydrogen into helium.
Assume
- The Sun was originally
100% hydrogen.
- The Sun can only
convert the
inner 10% into helium.
How much energy is that?
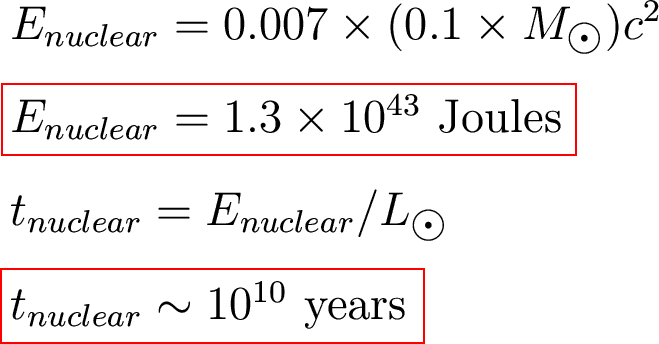 Oooh, now we're cooking...
Oooh, now we're cooking...
Nothing is free -- what's the problem
with fusing
hydrogen nuclei?
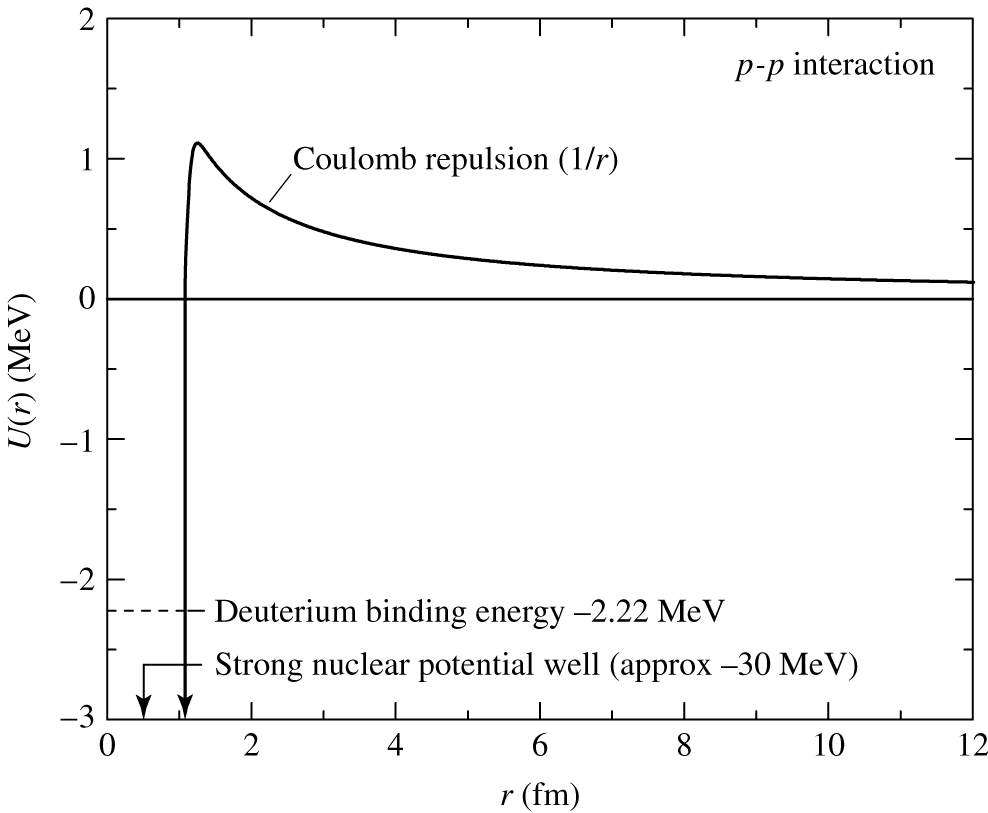
There are two forces acting inside atoms:
- The electromagnetic
force
- Long range
- Coulomb repulsion/attraction
- The strong nuclear
force
- Very short range
- Holds the nucleons (protons + neutrons)
together
(what are the other two
fundamental forces?)
Protons have positive charge. Like charges repel
-- the electromagnetic force.
We need to overcome this repulsion to have
the nuclei
fuse.
How do we do this? Energetic
nuclei!
How do we make energetic nuclei?


 Einstein into the picture. Einstein realized that E=mc2. Mass
and energy are equivalent, related by the speed of light, c.
Einstein into the picture. Einstein realized that E=mc2. Mass
and energy are equivalent, related by the speed of light, c.